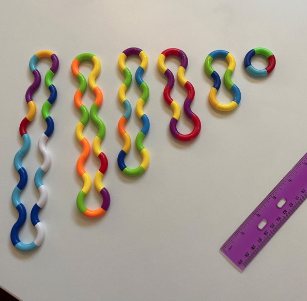On pure math in high school

In a previous post, On applied math in high school, I observed that the distinction between applied and pure mathematics is rarely discussed in the high school context. While that post focussed on applied math at VISST, in this instalment I will share my path to including pure math in the VISST curriculum.

My journey started with A Mathematician’s Lament, a piercing and influential essay/rant about the state of high school mathematics. The “Lament” was written by mathematician-turned-teacher Paul Lockhart in 2002 (and was later expanded into a short book). You may enjoy reading the first page about a musician waking up from a terrible nightmare; this is Lockhart’s metaphor for his own living nightmare as a mathematician. In the essay, Lockhart points out that few people understand what math really is: math is art, full of elegance and beauty, done for its own sake rather than practical gains. He (quite rightly) blasts high school math for being uninspiring, dry, and a terrible missed opportunity.

Fast-forward to 2022, my first semester of teaching math at VISST. I couldn’t help the feeling that something was missing, so I re-read Lockhart for inspiration. I asked my Grade 9 students to read it too and brainstorm with me, but I still lacked direction. Then, I encountered a podcast episode in which host Steven Levitt discusses math education with renowned mathematician Steven Strogatz. (I’ll take this opportunity to recommend one of Strogatz’s popular math books, The Joy of x.) Strogatz echoed Lockhart in lamenting that “people don’t have any idea what math is.” On the podcast, he proposes a high school “math appreciation” program, analogous to music appreciation or art appreciation classes, in which students learn to appreciate the beauty of mathematical ideas. It clicked. The following week, I hosted my first math appreciation class at VISST.

Following Strogatz’s lead, I based math appreciation on Discovering the Art of Mathematics, a set of 11 free books containing inquiry-based mathematics lessons. Each book is centred on a theme such as “The Infinite” or “Art & Sculpture” or “Patterns” or “Knot Theory”. The books don’t contain an “answer key” because such a notion would not be appropriate here; math appreciation activities are open-ended, but the books provide just enough scaffolding to send you sailing in the right direction.

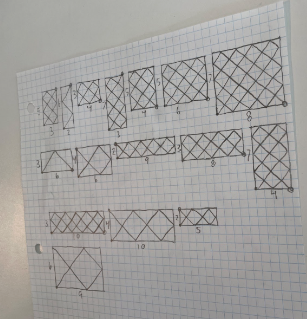
Here are a few photos from math appreciation at VISST in 2022-2023. As part of “Patterns”, students looked for patterns in a simplified model of a billiards game by drawing different trajectories of the “ball” and observing the results:

Some students progressed further than others through the activity, but it did not matter; no one reached the endpoint because it did not exist. We all simply enjoyed the “aha!” moments along the way. 

Here is another instance, this time from the “Knot Theory” book:

A common theme in math appreciation is the involvement of something tangible, whether it’s drawing patterns on paper or manipulating “tangle” toys (shown above) to construct knots. We concluded this particular activity by recreating the knots from the fantastic video How Knot to Hang a Painting. This formed a nice connection to physical reality. 

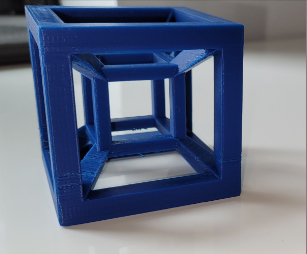
Finally, below are photos from a larger inquiry project that spanned several months in math class. Here, a student 3D-printed 3D representations a 4-dimensional hypercube by following along with a book, Visualizing Mathematics with 3D Printing:

Following the prints, the student was able to reason about how a cube’s various properties, such as the volume or number of edges, changes in different dimensions. 

Lockhart writes about math that, “There’s no ulterior practical purpose here. I’m just playing. That’s what math is— wondering, playing, amusing yourself with your imagination.” Because our math appreciation activities are inquiry-based, the students create the landscape as they explore, so no boundary or limit constrains them. Students are indeed exploring mathematics while playing. Unfortunately, the word “play” hardly feels apt when thinking about most high school math classrooms!

Making math playful and fun is beneficial even beyond the enjoyment of the moment. We all know that math often induces dread or anxiety in students (and adults, for that matter). I believe these positive mathematical experiences serve to strengthen students’ relationship with mathematics in general. This is so important, especially in cases where these feelings are complex or negative.

Looking ahead to the coming year, I hope to add more explicit connections between math appreciation and “regular” (or “applied”) math class, which I believe will benefit both aspects of the course. For example, the billiards game activity, shown above, ties in elegantly with ideas about proportionality, divisors and prime numbers. I also hope to introduce the idea of mathematical proof, and building appreciation for elegant proofs, which are often compared to poetry. And speaking of poetry, I am also keen to further explore the connections between math and literature and other arts. (If this sounds intriguing, you may enjoy reading Once Upon a Prime: The Wondrous Connections Between Mathematics and Literature.)

Embedded in our society is a deep misunderstanding of math. It is viewed as a tool based on arbitrary rules, or a particular type of hoop-jumping exercise, rather than a deeply creative art with a strong sense of aesthetics. In being intentional about making applied math genuinely applied, I wish for my students to wield math as a powerful tool at their disposal. In including pure math at VISST, I wish for my students to finish high school with an idea of what math is really all about. I wish for them to think of mathematical beauty with fondness — and, indeed, with appreciation.

Thank you to Edwin Yau for sending the podcast interview my way, and ensuing conversations.

If you enjoyed this post, consider joining the VISST mailing list at visst.ca/#updates!